[Originally published on CR-ministries.org]
Another Proof for God’s Existence!
Have you ever heard of the indirect proof for God? It involves a contrapositive.
What’s that?
Here’s a simple example: Statement A says, “If it rains outside for 10 minutes, then our grass is wet.” The contrapositive, Statement B, says, “If our grass is not wet, then it has not been raining outside for 10 minutes.”
Both statements go hand-in-hand. If one is true, the other is true. The contrapositive switches the hypothesis (if) and conclusion (then), by negating both at the same time.
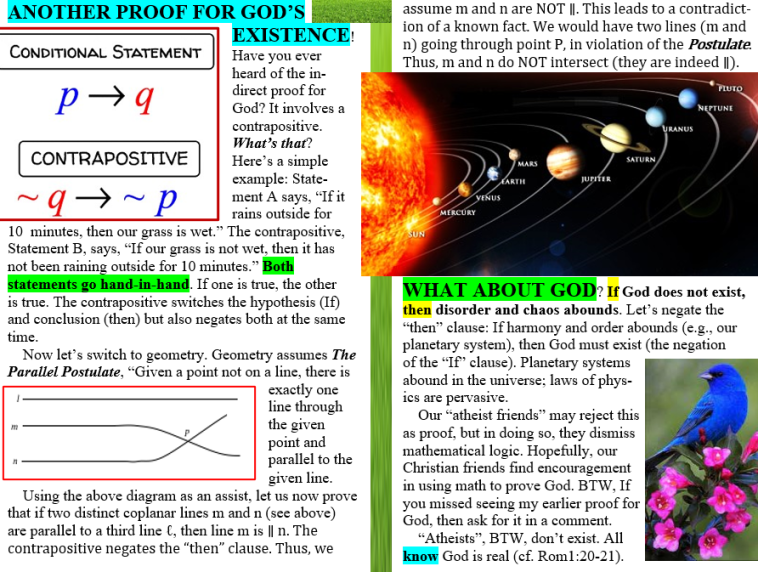
Now, let’s switch to geometry. Geometry assumes the Parallel Postulate, “Given a point not on a line, there is exactly one line through the given point and parallel to the given line. Using the above diagram as an assist, let us now prove that if two distinct coplanar lines m and n are parallel to a third line, l, then line m is ∥ n. The contrapositive negates the “then” clause. Thus, we assume m and n are not ∥. This leads to a contradiction of a known fact. We would have two lines (m and n) going through point P in violations of the Postulate. Thus, m and n do not intersect (they are indeed ∥).
What About God?
If God does not exist, then disorder and chaos abound. Let’s negate the “then” clause: If harmony and order abound (e.g., our planetary system), then God must exist (the negation of the “if” clause). Planetary systems abound in the universe; laws of physics are pervasive.
Our atheist friends may reject this as proof, but in doing so, they dismiss mathematical logic. Hopefully, our Christian friends find encouragement in using math to prove God.